Continuum Generation
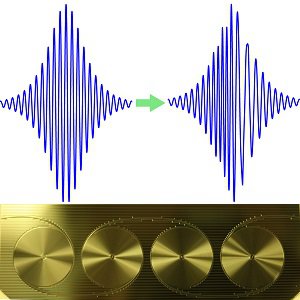
One application of the ULL waveguides that our group studies is continuum generation. Here, continuum refers to a spectrally broad optical signal - often over an octave in spectral extent. Continuum generation is used in optical frequency combs to broaden a mode-locked laser signal to enable implementation of "self-referencing". Continuum generation is readily accomplished in optical fibers [1] and there has been increasing interest in performing the process in a chip-based waveguide to potentially augment frequency microcomb performance or to enable continuum generation in wavelength bands that are difficult to access using optical fiber. The lower image in figure 1 illustrates a ULL waveguide that has been used in our group to generate octave-span continuum spectra [2]. The upper image illustrates how an optical field that is formed into a pulse is transformed by propagation through the waveguide. This transformation is caused by the Kerr-effect (the same nonlinearity used in frequency microcombs) to induce what is called "self-phase modulation" in the optical pulse.
The Kerr effect is a weak optical nonlinearity that produces a change in refractive index in response to a local optical intensity. An intense optical pulse will accordingly experience different refractive indices depending upon the exact location in the pulse. Accordingly, as the pulse propagates through the waveguide, the phase velocity will be different at different points along the pulse. As the pulse propagates it will acquire a phase shift that varies with respect to the envelope of the pulse intensity. This idea is illustated in the emerging pulse at the upper right in figure 1. The effect of this phase shift is to frequency-chirp the pulse. If the pulse intensity and the waveguide propagation length are large enough, the chirping will induce large spectral broadening.
The Kerr effect is a weak optical nonlinearity that produces a change in refractive index in response to a local optical intensity. An intense optical pulse will accordingly experience different refractive indices depending upon the exact location in the pulse. Accordingly, as the pulse propagates through the waveguide, the phase velocity will be different at different points along the pulse. As the pulse propagates it will acquire a phase shift that varies with respect to the envelope of the pulse intensity. This idea is illustated in the emerging pulse at the upper right in figure 1. The effect of this phase shift is to frequency-chirp the pulse. If the pulse intensity and the waveguide propagation length are large enough, the chirping will induce large spectral broadening.
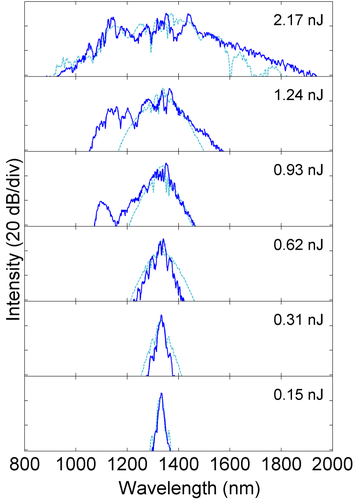
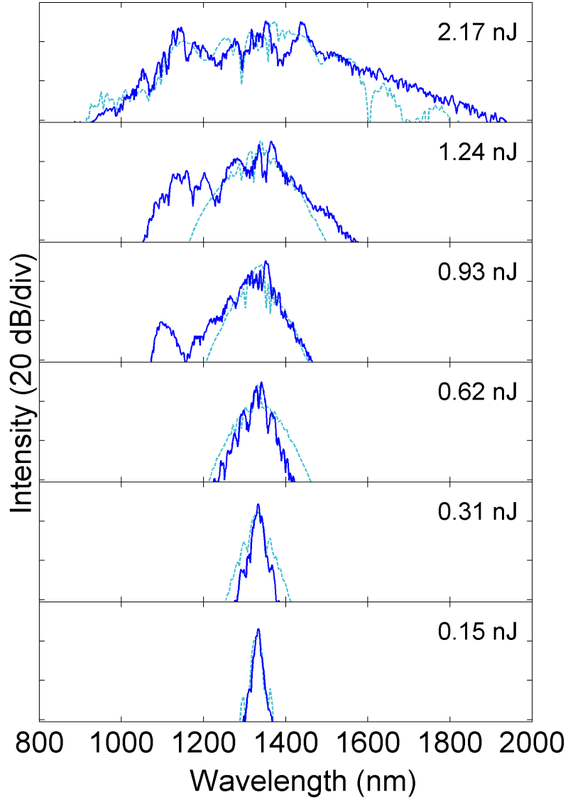
Figure 2 shows continuum-generation spectral data collected using the cascaded spiral waveguide in figure 1. Beginning at the bottom panel in the figure, the emerging spectrum correspond to pulses that have an energy of 0.15 nJ. In each subsequent spectrum, the pulse energy is increased. As this happens, the Kerr-effect in the silica waveguide combines with the pulse propagation (in this case over a length of 4 meters) to induce chirping of the initial pulse. In the upper panel, the pulse energy has been increased to 2.17 nJ and the spectrum extends over an entire octave of frequencies. We are interested in further improving this process by design of waveguides that better match dispersion to optimally maximize the effect of the Kerr nonlinearity. For this purpose, we are modeling the nonlinear beam propagation numerically. The light blue curve in each panel is the prediction from this numerical model.
- J. K. Ranka, R. S. Windeler, and A. J. Stentz, "Visible continuum generation in air-silica microstructure optical fibers with anomalous dispersion at 800 nm," Opt. Lett. 25, 25–27 (2000)
- D. Oh, D. Sell, H. Lee, K. Yang, S. A. Diddams, and K. J. Vahala, "Supercontinuum generation in an on-chip silica waveguide," Opt. Lett. 39, 1046 (2014)