Microwave Photonics
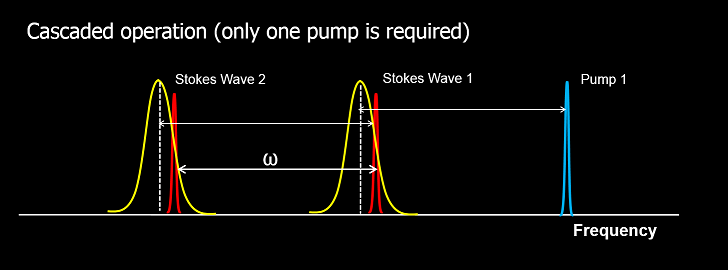
As background to this section, it is helpful to first read the research tutorial on Brillouin lasers. Referring to the diagram below, one interesting property of Brillouin lasers is their ability to "cascade" - meaning that once a Stokes wave achieves a certain optical power, this wave can, itself, act as a pump wave for another Stokes wave. The case of two Stokes waves is illustrated in figure 1. As noted in the section on Brillouin lasers, the resonator high-Q physics also causes the linewidth of the individual Stokes waves (i.e., their full-width-half-maximum spectral width) to be very narrow - less than 1 Hz. However, even with this very narrow linewidth, the center frequency of the cavity modes on which these laser lines oscillate will fluctuate as a result of temperature changes and acoustical noise from the environment. Nonetheless, because the two Stokes waves exist in the same optical resonator these environmental disturbances largely affect each of the Stokes waves identically.
Figure 1: Cascaded Brillouin laser action. The difference frequecy of the Stokes laser fields is stable and used to generate microwaves.
As an anology, imagine that two people travel on a bumpy road. If the people are traveling in separate cars, then their bodies will move somewhat independently in response to the bumps in the road. However, if they travel together in the same car, then their body motions will be highly correlated. The correlated motion of optical frequencies within the same resonator can be used to advantage in the cascaded Brillouin laser by detecting the difference frequency of the two, Stokes waves. Detection here means that we simply couple the waves using an optical fiber onto a photo detector. The photodetector converts optical power into an electrical current by squaring the total incident electric field. This "square-law" process produces a component of photo current at the difference frequency of the two Stokes waves. This oscillating electric current is very stable, because the Stokes waves share the same resonator (environmental noise is suppressed) and because each Stokes wave is spectrally very narrow (physics of laser action in a high-Q resonator).
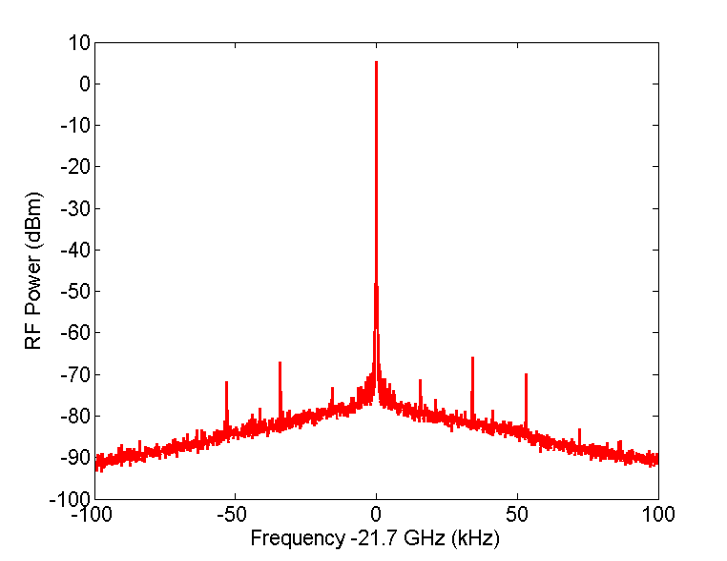
As a result, the cascaded Brillouin laser can be used to produce a spectrally pure electrical signal at the difference frequency of the Stokes waves. For neighboring Stokes waves, this difference frequency is about 10.8 GHz, which is called an X-band microwave signal. However, we can also allow the cascade process to proceed to even higher orders and detect Stokes waves that are multiples of this value. One particularly useful pairing turns out to be the first and third Stokes waves. These are 21.7 GHz apart and also happen to propagate in the same direction - making them easy to detect. The spectral beat produced by this detection can be analyzed using an electrical spectrum analyzer. The beat from Stokes 1 and Stokes 3 wave in the synthesizer (see figure 3) is shown in the figure to the right.
Figure 2: Stokes 1 and 3 Spectral beat.
By tuning the optical frequency of the blue pump wave in figure 1, it is possible to make a fine adjustment to the frequency difference of the two Stokes waves and therfore also the X-band microwave signal produced by their detection. If we were to consider the entire cascaded Brillouin laser as a black box with electrical tuning control input to the pump at one end and X-band microwave signal at the output, then the black-box device would be called a VCO (voltage controlled oscillator) by any microwave engineer. VCOs are extremely important devices that are found in communications systems, electrical test equipment, radars and in navigation systems. In this case, the VCO is all optical. However, we can still treat it like an electrical VCO because it has an electrical input and output.
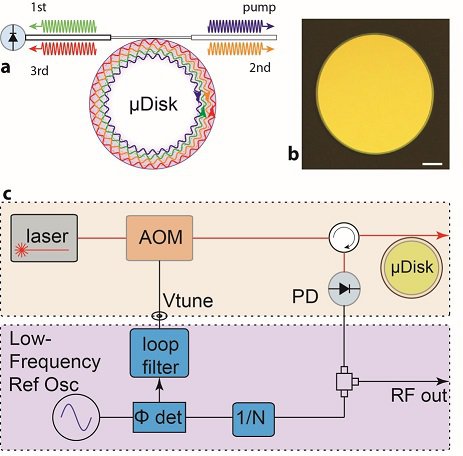
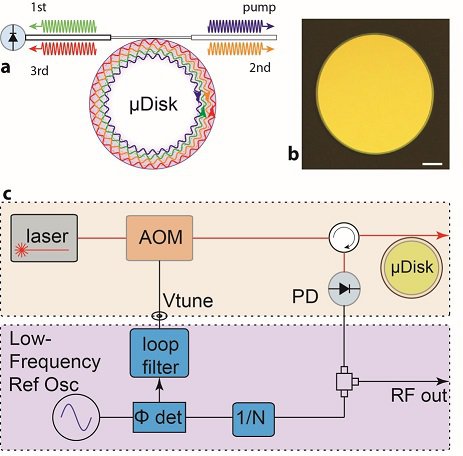
Figure 3: Synthesizer with an optical VCO
In the panel at the left is shown a circuit diagram for a device called an electrical frequency synthesizer [1]. Electrical frequency synthesizers allow us to generate almost any desired sinusoidal signal as an electrical current. They work by connecting a VCO to device called a frequency divider (shown in the diagram as "1/N"). The divider accomplishes two things. First, it allows generation of new signal frequencies that are lower than the VCO frequency. Second, The frequency divider divides the high frequency VCO signal down to a frequency low enough to be compared to a quartz-crystal oscillator (currently one of the most stable electrical oscillators). This comparison is used to generate a correction signal that tunes the VCO so as to further stabilize its frequency. We have repeated this same process except using the Brillouin laser VCO. The result is a kind of optical-based synthesizer [1]. In the research section entitled electro-Optical Frequency Division, this idea is taken a step further by flipping the architecture of the synthesizer and replacing the quartz with an all optical reference and the electrical divider with an optical divider.
Jiang Li, Hansuek Lee and Kerry J. Vahala, "Microwave synthesizer using an on-chip Brillouin oscillator," Nature Communications 4, 2097 (2013)