Nonlinear Resonators

UHQ resonators enable light to build-up in power in a small volume. A milliWatt of optical power can be magnified to over a kiloWatt of continous, circulating power. This makes possible access to nonlinear physics at remarkably low power levels. In this section, a brief introduction to this behavior is given in the context of third harmonic generation. Many of the applications under study in our group use a host of other nonlinear phenomena to control and manipulate light.

Intuitively, one can imagine that a whispering gallery resonator is like a traffic-round-about except with photons replacing the automobiles. In this analogy, high-Q resonators would be very inefficient round-abouts in which the probably of exiting each round trip becomes smaller and smaller as the resonator Q factor increases. In such a situation, one could imagine that a small trickle of incoming photons would lead to a large number of photons trapped in the resonator round-about. This would, in turn, cause traffic jams with only a small input rate of photons. As it turns out, these photonic traffic jams are both interesting and very usefu and they provide one way to apply resonator, high-Q physics.

Figure 1: Third harmonic generation.

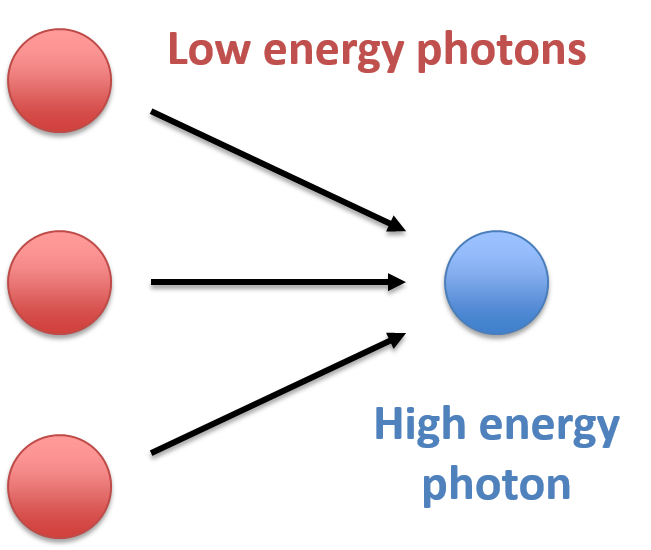
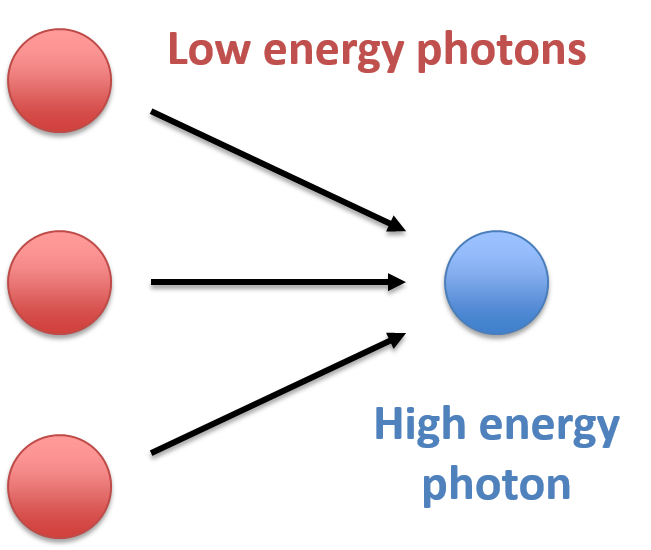
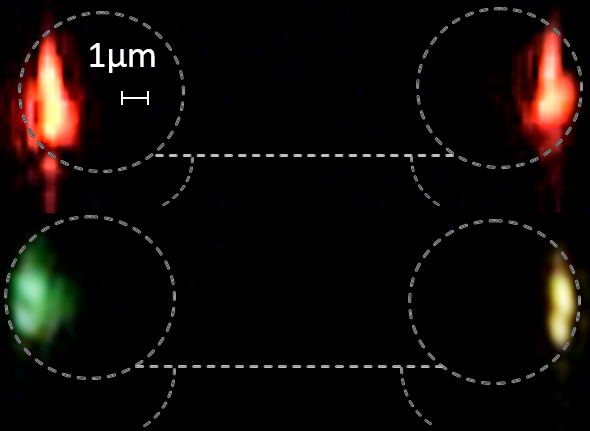
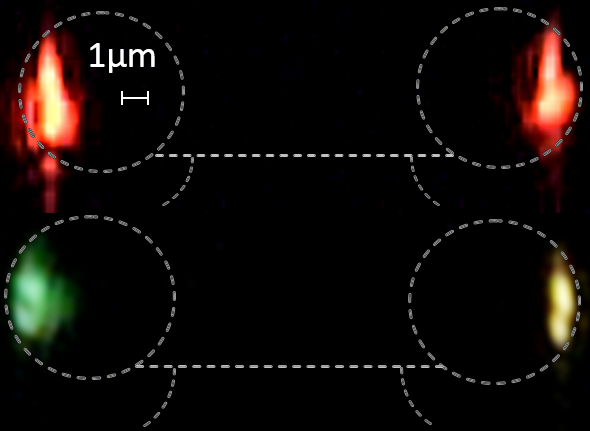
The photonic realization of a traffic jam is optical nonlinearity. Photons very weakly interact in materials, however, when concentrated in a high-Q resonator their collisions become more and more likely. Eventually, a weak input of photons to a small, high-Q resonator causes frequent collisions (i.e., strong nonlinearities). For example, one kind of nonlinearity that we have studied involves the collision of three photons. These photons fuse together through a nonlinear interaction called third-harmonic generation and create a single new photon (Figure 1). Through energy conservation this new photon has three times the energy of the colliding photons and therefore three times their frequency. This means, for example, that three infrared photons with wavelengths around 1.5 microns (not visible to humans) will fuse together to form a visible photon with a wavelength near 0.5 microns. In fact, by tuning the frequency of the invisible photons one can generate a whole spectrum of colors.Two measurements of third harmonic generation are presented in figure 2. In the upper-half of the figure orangish-red light is being generated by infrared photons trapped inside a microtoroid resonator (dashed lines give the outline of the microtoroid). Also, in the lower half of that figure, two frequencies of infrared light are introduced in the microtoroid. Curiously, these are circulating in opposite directions in the round-about microtoroid so that we see two distinct colors (yellow and green) emerging as a result of the collosions. It is also fun to realize that unlike an actual round about, the colliding photons that enable the generation of the green and yellow light in each direction are magically able to pass through eachother when propagating in opposite directions. This feature results from the wave nature of the photons and is not captured by our simple traffic analogy. More details on this experiment was performed can be found in reference 1.

Figure 2: Measurement of third harmonic generation.

Another kind of photonic collision is more of fender bender in that two photons collide and simply exhange energy and momentum (as opposed to fusing together as in the example above). This particular interaction leads to a phenomena called parametric oscillation which our group was first to observe in microcavities [2]. Also, when the collisions cascade, as is easily arranged in a resonator, the compounding fender benders produce optical frequency combs. Frequency combs are an active area of research in our group.

Sometimes the high concentration of photons in the resonator enables interactions with phonons. As one example, the subject of cavity optomechanics, whose name we coined, involves interactions between lightwave and radio-frequency sound waves. Also, a class of laser that we are currently studying, the Brillouin laser, uses microwave-rate phonons to create highly coherent lightwaves and even electrical microwaves. These areas are described in more detail in other parts of this website.

T. Carmon and K. J. Vahala, "Visible continuous emission from a silica microphotonic device by third harmonic generation," Nature Physics, 3, 470 (2007).
T. J. Kippenberg, S. M. Spillane, and K. J. Vahala, "Kerr-nonlinearity optical parametric oscillation in an ultrahigh-Q toroid microcavity," Phys. Rev. Lett. 93, 083904 (2004)